In my inventory is a table-size (9 feet by 5 feet) map containing 8 regions, 8 "countries", each of which is divided into 6 provinces. For purposes of the movement of forces around the map, each province is, in turn, divided into 2 "counties". The result is that each county is around 8 inches square, sufficient to hold a couple of 2"x2" inch stands in a large area-map game..
Also in inventory is a huge quantity of plastic figures... red plastic and blue plastic, infantry and cavalry and artillery, which measure approximately 20mm in size. They are mounted on 2x2 stands, and the number of figures on each stand varies, from 4 to a huge infantry behemoth of 12 figures.
Sad to say, these figures have never been painted... they thus retain their untouched, environmentally pristine nature, but it does make it easy to discern which army is red, and which is blue.
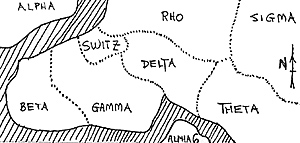For this adventure, the Alphans, located in the north-west corner of the map, were pitted against the Sigmoids, far to the east. Note that Sigma has no access to the sea, and it’s easy to see that Sigma’s desire is both to strike to the west and to gain a sea port or two. Note also that Alpha, isolated from the mainland, is by definition, a sea faring country and has an outpost province, Alpha6, located in the south.
In essence, the map faintly resembles Europe... England stands in the north-west corner, separated from the continent by the Channel, with Russia far out in the east. But it’s not Europe... it has nothing to do with Europe, and I refuse to acknowledge any similarity.
When Bob Liebl, who participated in a game, started to say "It’s obvious that England, as a sea power... ", I immediately shushed him... "What England?", I said, "I don’t see any England! I see only Alpha and its neighbors. Let’s not confuse ourselves with historical reality."
For the big game, both Alpha and Sigma were each given around 10 infantry stands (1 stand was defined as an army), and 7 cavalry stands. Each army had a combat strength composed of two factors.
- First, there were the number of figures on the stand, N.
Second, each was assigned a strength value, S, which started at 4, and which decreased as a result of the army engaging in combat. The S factor could also be increased by devoting resource points to the army. If the S factor went to zero, the stand was removed. There were no reserves in the campaign
The total combat strength of the army-stand was the sum of N + S, where N was a constant and S was a variable.
Cliff Sayre and I engaged in the first test of the rules system... I was the Sigmoidian commander, while Cliff set up shop in Alpha. Both of us started with no units on the field... we each then diced, when our side was active, to see how many units (stands of infantry or cavalry) appeared, and this could turn out to be 4, or 3, or 2 stands. These stands appeared in the capitals of our respective countries.
Then, after our units appeared, we again diced to see how many groups on the field could be activated to move and engage in combat. Here, the result was either ‘all of them’, or ‘all but 1 of them’, or ‘all but 2 of them’. A group of units within an area was termed an "army" and it could be composed of a general, an artillery piece, and two combat stands (either infantry or cavalry). This group of 4 was defined as the ‘stacking limit’ for every area.
As the Great Leader of Sigma, my dicing was horrible... first, each time I determined how many units appeared, only two of them materialized. And second, when I diced to see how many of the existing groups on the field could move, the result seemed to be ‘all but 2 of them’.
In contrast, Cliff always seemed to be able to get the maximum number of units to appear and to move his entire force around (an ‘all of them’ result).
I had thought that Alpha would be greatly disadvantaged, since her troops had to appear in Alpha proper, and then they had to board transports, and then they had to sail across the Channel, and then they had to disembark to start their advance across the mainland. But due to Cliff’s fine dicing efforts... compared to my own rotten efforts... he managed to get a huge jump on the Sigmoidian forces, capturing a host of key towns, termed ‘resource areas’.
Resources provided points, and points could be used to augment a stand’s strength, S, factor. There were some ten resource areas on the map, and after the Alphans had captured most of them, we quit... a resounding Alphan victory. My forces couldn’t seem to get out of the gate.
But the next day, I hosted a bigger, better (?) game... 5 living, breathing people at table-side. Bob and Cleo Liebl for Sigma, versus the Alphan crew of Stephan Patejek, plus Jim and Pat Byrne. And, of course, due to the problems arising during the first game, I had changed the rules. All was to run calmly and serenely... of that, I had no doubt. Each side was given an assortment of generals... and each general was graded. An "80" general had an 80 percent chance of moving the forces within his area when his turn came, a "70" general could get his troops moving with a 70 percent chance, and the lowest of the low, a "60" general (last in his class at the Point), had only a 60 percent chance to go active.
In this fashion, I got rid of the table that had listed "all groups move", and "all but one group moves" and so on. This time, each individual general had to be diced for. Which meant that where the going was critical, the sides placed their "80" generals to ensure their armies would keep moving.
I did provide a capability of having unescorted units move, i.e., there didn’t have to be a general in an area to get a unit moving... an isolated stand could get going with a 50 percent probability. But 50 percent wasn’t too reliable a percentage, as Cleo Liebl found out. She had sent out a couple of cavalry armies, unescorted, and over half the time, they simply refused to budge.
Alpha was given 5 transports with which to get her troops to the mainland. she started out with one, and each turn, another one of the ships appeared. A transport could carry an "army", that is, a general, a battery, and 2 troop stands. This meant that Alpha had to sail the transports to the mainland, disembark the troops and then sail back for another load... too, too slow.
Key Change
One key change that I made to the system concerned the method of tracking unit (stand) losses. In the first effort, when Cliff and I faced each other, each stand was tracked for its basic strength, termed S... this was a number ranging from 4 to 8. Each time the stand took a hit, the S factor was reduced. When S reached zero, the stand was removed.
The melee procedure took into account both the number of figures on the stand and its strength, S, giving it a total combat value, which, in turn, gave it a striking capability.
In the second game, the tracking procedure was reversed. This time, instead of being concerned with strength, we tracked a stand’s losses, i.e., hits. When a stand reached a total of 5 hits, that signified destruction.
The resource areas provided points with which, in the first game, we wanted to raise the strength value. In the second game, the resource points provided a capability of reducing the recorded loss value... two different methods of achieving the same final result.
Combat System
The combat system itself, consisted of 5 phases, starting when a moving force entered an enemy-occupied area.
- (a) Any artillery on either side would fire with a basic probability-of-hit (POH) of 50 percent. If a hit was scored, the target force was given a casualty marker... the marker was not assigned to any specific stand.
(b) Then the cavalry of both sides struck. Each stand had a base of 30 percentage points. To this, was added 7 points for every figure (N) on the cavalry base. The chance to strike was thus POH for cavalry = 30 + (7 x N) Again, casualty figures were given if a successful strike occurred, with the markers not assigned to any specific stand.
(c) After the cavalry struck, each cavalry stand would be diced for... there was a 70 percent chance it would hang in there for the grand slaughter and help out when the infantry attacked. If the cavalry failed to stick around, it pulled back and watched the infantry go in.
(d) Now, the infantry went in, assisted by any cavalry that had decided to help out. The POH for the cavalry was the same as that given in step (b), above. The infantry stands had the same base of 30 percent, but each figure on the base was worth 3 points, vice the cavalry’s 7. POH for infantry = 30 + (3 x N)
(e) Each side totaled all its figures (infantry and cavalry), added the casualty figures it had inflicted on the opposition, multiplied them by a factor and tossed in a percentage dice throw to determine the winner. Each side then allocated the casualty markers it had received to specific stands, keeping in mind that 5 hits knocked off a stand.
Bob’s and Cleo’s Sigmoidian forces overran the field, capturing every resource area but one, at which point the Alphans finally threw in the towel. Our post-game discussion looked at the ‘unbalancement’ that caused the one-sided result.
The key cause of the unbalance in the system stemmed from the combat results table. In combat, the units within an area were pitted against each other. Each area could hold a battery, and 2 stands, either of infantry or cavalry. All struck at the opposing force, with a successful strike resulting in the opposition receiving a casualty figure. Then, to determine the winner, a quick count of the total number of figures involved, plus the number of casualty figures on the opposition, plus a dice throw, gave the result.
The actual combat calculations and the determination of the winning side were not at issue. The critical factor here, was the final disposition of the losing force. While the winning force held position, retaining control of the disputed area, the entire losing force was picked up and immediately "wafted" back to its capital. There, it recuperated and could be sent out again.
This meant that if a Sigmoidian army lost a battle, it went back to Sigma proper, and could rapidly get under way again. In contrast, if an Alphan force lost, it would whiz way, way back across the sea to Alpha, and there, to get into battle again, it would have to board a transport, sail across the Channel, and disembark before it could advance.
This delay was what caused Alpha to lose so disproportionately. Alpha was placed at a huge disadvantage in getting her forces back in action on the mainland.
Several ‘antidotes’ were looked at. One was to give Alpha an initial foothold on the mainland at the beginning of the game. Another was to provide Alpha with an infinite supply of boats, so that she didn’t have to rely solely on her 5-ship transport fleet. Another was to have a losing force retreat to a friendly resource center, instead of making a mad homeward dash for its capital.
How I’ll eventually amend the rules, only time will tell.
Back to PW Review May 2001 Table of Contents
Back to PW Review List of Issues
Back to MagWeb Master Magazine List
© Copyright 2001 Wally Simon
This article appears in MagWeb (Magazine Web) on the Internet World Wide Web.
Other military history articles and gaming articles are available at http://www.magweb.com