Selecting a ground scale for the wargame is not the straightforward matter of 'adopting' any mathematically-easy to operate one that rulemakers appear to think. The wargames table is, in effect, nothing more than a 'map' of the area over which the battle is to be fought, with certain of the more prominent and tactical ly-important features shown in relief, and this is a factor which must be borne in mind by the rulemaker when determining his ground scale, and from that his figure scales. He must, for example, keep in mind that on his wargames table (map) he is required to employ certain areas - the bases of the figures - whose actual dimensions will not alter, but which are required to represent specific dimensions on the ground under his ground scale. To illustrate the problem facing rulemakers we may take the extreme, but nevertheless valid, example of a wargame played over a map of Waterloo on a 1 inch to 1 mile ground scale using 25mm figures, the infantry base dimensions of which are of the order of 10mm by 16mm. If we apply the scale to the length of the figure's front (ie standard wargames practice) we will find that the figure's base is 704 yards long. Using standard wargames practice therefore, we would say that the figure represented a division of 6 or 7 battalions in line and that would be the end of the matter. However, the depth of the figure under these circumstances would represent a distance of 1126 yards, (some 1/3 of a mile), which is more than the depth of the entire French army at the battle of Waterloo. Clearly, such a state of affairs would be entirely devoid of the realistic representation of the conditions of the period.We can see that, in order that the base size of the figure can be made to equate with the map (and since we cannot change the figure) we must use a map whose ground scale is such that it will cause the base dimensions of the figure to represent what they are supposed to represent - namely the depth, and part of the length of, the base linear formation. After all, all formations are combinations of the linear formation! The example, although extreme is illustrative of the situation currently existing in wargaming. As an instance of its effect in the game, we need look no further than the commonly employed combination of 25mm figures with a ground scale of 1 mm:1 yd. The dimensions of the infantry figure base represents an area 10 yards wide by 16 yards deep. A line of 20 figures, therefore, will represent a battalion of infantry with a frontage of 200 yards and a depth of 16 yards. Under the conditions of the Napoleonic period the depth of the three- deep line was only 4 yards. Thus we must arnmend our map scale (or ground scale employed on our table) to cause the figure's base to meet the dimensional requirements of the basic line of battle for the period - it follows that the correct ground scale for our games is, in this instance, one of 4mm:1 yd. We can see that the failure to consider the effect of their ground scales on the dimensions of the figure's base has had an adverse effect on the accuracy of the game. This effect increases as the model formation becomes deeper - a simpler line being 12 yards 'too deep', but a column of four companies, with a true depth of 18 yards, being no less than 64 yards deep in scale. What we do when we do not take the ground scale's effect on the dimensions of the figure's base into account is cause one part of our representation of reality to operate at a different scale from the remainder. This is a vitally important point to wargamers as, it follows, that measurements made on our tables will be on different scales depending on the position in the unit to which the measurement is made. Using our first example of a 1 inch to 1 mile map, we can immediately see that a measurement from a position in front of the figure to another figure immediately behind it, (which would, in reality, be 4 yards behind the front of the first figure), would be taken by us as being almost 1/3 of a mile from the front of the first figure, as shown in Sketch 1. A In reality 4 yards, measured as 1126 yds making range B 1122 yards too long in terms of what the figures represent Oe two lines of infantry, the second 4 yards behind the front of the first). We are, regardless of how we look at the problem, faced with the fact that wargames are currently being played out within an infinity of different ground scales operating simultaneously in the one game. 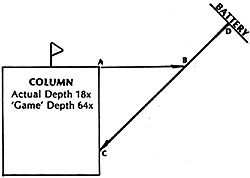We may see the truth of this assertion by considering the measurement being made in Sketch 2, where a battery of guns is having its range from a column of infantry determined. Since the depth of the column is excessive in relation to what it would be in reality, the length of the line B-C is greater than it would have been in reality, and that, consequently, the length of the 'range' measurement, D-C, is also greater than it ought to be. We can also see that the ,error factor' is not the whole of the line B-C, but that part of it 'added on' to cope with the fact that the line A-C has been 'elongated'. Surprisingly, there is no great problem in determining the proper ground scale for use with any size of figure and any period of history. All that we need do is calculate the ground scale that will cause the depth of the figure to equate to the depth of the basic linear order of battle. Of course, employing such a system to 'find' our ground scale(s) will have an effect on our figure scales, since the latter are derived from the former. Let us, therefore, consider the effects that the use of the proper procedure for determining the ground scale will have on our figure scales in perhaps the two most popular wargaming periods, the Napoleonic and the Ancient. We saw that the depth of the figure was required to equate to a distance of 4 yards under the conditions of the Napoleonic period, and that a ground scale of 4mm: lyd is required. The front of the figure, some 10mms long, would equal 21/2 yards, or that occupied by some 4 men (using the'official' regulation width of 22 inches per man) a figure scale of 1:12. This requires us to deploy 50 figures in single rank to represent the common wargames battalion of 600 men. As such a battalion would have table dimensions of 500mms (11/2 feet) by 16mms (5/8 inch), we would, obviously, not get very many on the average wargames table of 6 feet by 4 feet representing an area 4571/2 yds wide and 305 yds deep. There are two ways in which we can modify the ground scale without losing any of the accuracy which we seek. The first of these is that, where there exists an interval behind our units which will not be occupied by other troops, we may include that interval within the depth of the figure. During the Napoleonic period even in close columns, a minimum of 3 yards was maintained between sub-units in the column. We may cause the depth of our figure to equate to a distance of 7 yards, including both the depth of the line and the depth of the interval behind it. All that this would mean, in terms of the visual aspect of our games, is that we would represent close columns by placing ranks of figures behind one another with their bases touching, in keeping with current practice. Thus our 16mm depth would give a ground scale of 2.28mm:1 yd, which we could render as 2mm:lyd by accepting the very minor degree of error, or could render as 2mm:1yd accurately by basing our figures onstands which were only 14mm deep and filing away 2mm from the front or rear of the figures. This would cause the f ront of the f igu re to represent 5 yards, or the frontage of some 8 men, and would only require us to deploy 25 figures to represent a 600-man battalion. The table dimensions of our model battalion would be reduced to 250mms (9ins) by 16mm (5/8 in). Our table area would now have dimensions of 915 yds by 610 yds. The principle forces of the Ancient period deployed, of course, in massive phalanxes, on a depth of many ranks, without the 'mathematical' precision of the Napoleonic regulations. If, for example, we allowed that the basic depth of formation during the period was 20 ranks, allowing similar dimensions 'per man' to those applicable during the other period, the depth of the figure would equate to a distance of 32 yards. This would provide us with a ground scale of 1mm:2yds, causing the front of the figure to equal 20 yards - or the frontage occupied by some 33 men, which, on a depth of 20 ranks, would give us a figure scale of 1:660. Obviously, such a figure scale, causing individual figures to equal units in most cases, and requiring relatively few figures to be employed on the table to represent Ancient armies, would be opposed to our need to be able to operate the tactics of the period, which require a greater degree of 'internal flexibility' than would be the case here. We can improve things by including the interval in the scaling of the figure base as described above. Since, ultimately, we are required to represent the depth of the basic formation accurately - although we may do so with a figure formation of more than one deep - it follows that whatever depth we require the figure to equate to must divide evenly into the basic depth. (A depth of 6 ranks, therefore, would not be sufficient, since this would require us to deploy formations 33 1/3 figures deep in order to represent phalanxes 20 ranks deep). Taking the basic phalanx to be 20 ranks deep, therefore, for example, we may require our figures to equate to the depth of 5 ranks of men, or some 8 yards, which would give us a ground scale of 2mm:1yd, causing the figure's front to equal 5 yards, or 8 men, and giving us a figure scale of 1:40. By this procedure, an army of 1320 men would be represented by 32 figures as opposed to the 2 figures which would have represented it under the other scale. The reader will have observed that wargaming is not well- served by the 25mm, scale of figure, and that, in order to make the game more playable, while retaining accuracy in our representation of our period's warfare, we must turn to the smaller scales of figures. For example, the base dimensions of those 15mm figures which the author has used are of the order of 6mm by 6mm, requiring us to operate with a ground scale of 1 mm:1 yd, (accurately by mounting the figures on stands 7mm deep), which causes the front of the figure to equal that occupied by 9.8 of 10), men. The figure scale therefore becomes one of 1:30 under Napoleonic conditions, requiring us to deploy only 20 figures to represent a 600-man battalion, which would have table dimensions of only 120mm (4 3/4 inches) by 7mms (1/4 inch), thus permitting us to employ far more such units than would be the case with 25mm figures. To equate the depth of the 15mm figure to the 8 yards depth of five ranks of Ancient infantry would require us to base our figures on stands 8mms deep for perfect accuracy, giving us a ground scale of 1mm:1yd, and a figure scale therefore, of 1:50. Since Ancient battles do not, as a general rule, require anything like the ground area to reproduce as do those of the Napoleonic period, we may reduce the figure scale by increasing the ground scale. Thus, if we were to require the depth of the figure to equate to the depth of only 2 1/2 ranks, or 4 yards, we would require a ground scale of 1 1/2 mm:lyd, which would mean the front of the figure representing the distance occupied by some 6 men, giving us a figure scale of 1:15. This would permit us to depict an army of some 10000 men with around 660 figures rather than the 200 figures required under the previous scale, although it would ,reduce' our table area from dimensions of 1830x1220 yards to dimensions of 1220x813 yards. The 1/300 scale figures employed by the writer and his colleagues, made by the British 'Heroics' company, have individual base dimension of 3 x 3 1/2mms. For the Napoleonic period, therefore, we would require a ground scale of 1 mm:2yds, giving a figure scale of 1:30, since the front of the figure would represent the front of 10 men, and requiring us to field 20 figures to represent a battalion of 600 men. Whilst this would be the same number of figures as when using 15mm, the table dimensions of the unit would only be 60mms (2 1/3 inches) by 3 1/2 (1/8 inch) in this case, permitting us to field even more units than before. For Ancients at a ground scale of 1 mm:1 yd, the depth of the 1/300 scale figure would represent 4 yards, or the depth of three ranks of infantry. The front of the figure representing 5 men in rank (a figure scale of 1:30) an army of 10,500 men would require 700 figures, an investment of $17.64. The table area for a 6x4 foot table would be 1830x1220 yds, which would give plenty of room for wide-flanking movements to get away from the usual 'head-on collisions'. Having determined the ground scale accurately from the principles given in this article, we would then use them in the same manner as at present to determine the figure scales for our mounted troops, for other infantry that was deployed in a different way from the normal formation, (eg 2 deep lines during the Napoleonic period), and for our artillery and, where applicable, animals such as elephants. Using an accurate ground scale would mean that where differences in deployment between troops with the same physical dimensions occurred, which were not reflected in the base dimensions of the figure, different figure scales would operate so that bases of the same size would indicate different figure/men ratios depending on the unit's deployment (2 ranks, skirmisher, etc.). As the purpose of this article, however, is merely to present the reader with the data required to determine the ground scale, the subject of individual figure scales will be retained for a future submission. Back to Table of Contents -- Courier Vol. III #4 To Courier List of Issues To MagWeb Master Magazine List © Copyright 1982 by The Courier Publishing Company. This article appears in MagWeb (Magazine Web) on the Internet World Wide Web. Other military history articles and gaming articles are available at http://www.magweb.com